
Losing to the Algorithm
Each year my 7th graders come to me with varying levels of number sense. I have many that show up in August feeling insecure about their ability to “do” multiplication and division. They feel defeated by the algorithms of their elementary school years. Either they weren’t “quick enough”, or they can’t remember all the specific steps, or it just never made any sense! It was a game of memorization and they felt like they had lost.
How do we fix it?
I spend the entire first month with my 7th graders showing them that they CAN multiply and divide by just THINKING deeply about the questions and using number patterns. They don’t have to have any previous knowledge about steps and procedures to be able to do so! After a month of showing them how this is done, I have 7th graders who are confident in their skills in these operations. So what do we do for that month? Lots and lots of Number Talks!
I like to start with this one question: “What is 18x5”? Then I give the students a few minutes to think about the problem in their head, and give a thumbs up when they have a solution ready. After about 2 minutes, every student has their thumb up. Then I have the students say the answer at the count of 3. 1…2…3… “90!” they all yell together. “Yes! That’s correct!” I say. “But that’s not the interesting part! The part I’m interested in is HOW you came up with that answer”. We then spend the rest of the class time sharing the various ways that the students THOUGHT about the question, in order to come up with an answer. Most of the strategies that the students use fall into one of 4 categories. And NONE of them are a standard algorithm (I didn’t even allow them to use paper). Pam Harris discusses these strategies in her podcast Math Is Figure-Out-Able, and I’m going to use some of her terminology here. (Check out her podcast for super great strategies in building number sense).
4 Strategies for Multiplication (that aren't algorithms!)
1) Partial Products
This is when the student keeps one number whole, but splits up the other into simpler
parts.
Examples: Keep the 5 and split the 18 into 10 and 8. 5x10=50, 5x8=40, so 50+40=90.
Keep the 5 and split the 18 into 9 and 9. 5x9=45 and 5x9=45, so 45+45=90

2) Over/Under
This is when they change one of the numbers to be a little bit bigger or smaller, in order to change the problem into a simpler one, but then adjust at the end.
For the problem 5x18 it would look like changing it to 5x20…. 5x20=100 but it’s two 5’s too much, so you move back to 90.

3) Doubling/Halving
This one is very interesting and extremely helpful. You can make an equivalent problem by halving the amount of groups, but making them twice as large. So in 5x18, instead of 18 groups of 5, you can instead have 9 groups of 10. 9x10=90 is a simpler problem to think about.
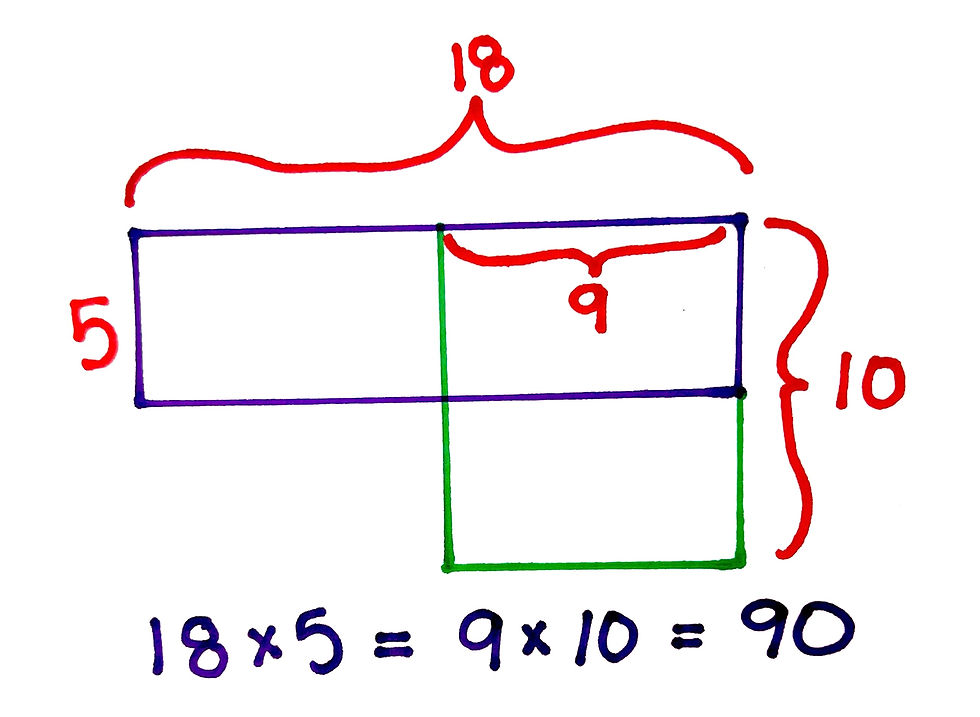
4) Five is Half of Ten
This strategy just requires students to think about “times 10” and then halve it to find out “times 5”. So instead of 5x18, the student can think of 10x18 which equals 180. Then take half… so 90.

Defeat the Algorithm, Not the Student
In my 5 years of prioritizing these multiplication strategies with my 7th graders, I've never had a student that didn't grow more confident, and less anxious, about math. Once a student discovers and believes that they are capable of doing math WITHOUT the need to memorize a series of long, boring, confusing steps, they're unstoppable. They become critical thinkers, creative problem solvers, and confident risk takers in the classroom. It's my favorite thing to see! Try asking your students "what is 18x5?" and see what creative strategies they come up with!

Comments